Physical Causality and Work
NEW 2020 (I am still working on this page)
What really lies behind causation?
On our causation page, we start with Aristotle, recognising enquiry into cause as at least partly a philosophical problem. In his account, causation involves four categories: material, efficient, formal, and ultimate (final cause), because they are the four natures (or aspects) of causation. Most modern philosophers pay little attention to this as by far the majority of their current work concerns efficient cause only, which is usually taken to be the only true cause (many believe the other three were not really causes at all). Efficient cause is the dynamic action of transformation, moving or converting one thing to another and it coincides with a rough `common sense' idea about causation.
Without rejecting other aspects of cause (see shortly), I adopt a physicalist view, which claims that in the physical (material) world, what we observe as efficient causation is always the action of a physical force (usually, but not necessarily on matter). More precisely, the physical mechanism behind cause is a transfer of a conserved quantity (energy, momentum or something more exotic like charge or spin) in a material system according to the transference theory of Salmon (1984) and Dowe (2000) which posits that there must be a spatio-temporally continuous connection between one thing X and another Y involving the transfer of energy, momentum (or other conserved quantity) for X to cause Y (the connection is via a force field). In other words, efficient cause reqires physical force, which is known to be the consequence of an exchange of some force-carrying particle (or wave).
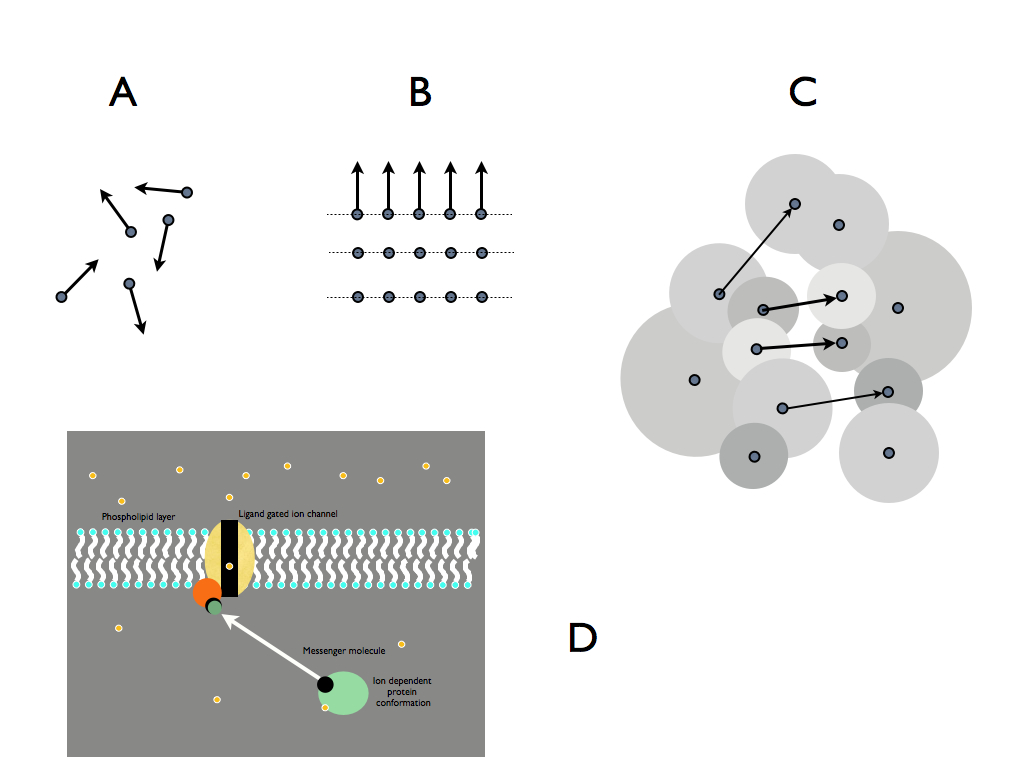
Physical forces all either cause movement or its prevention and all have an orientation (direction) in space. The realised movement (or prevention of it) is the vector sum of all the physical forces acting on a particle at one time. In the absence of constraints the vector sum of forces acting on each member of an assembly of particles is random and accordingly has no (ensemble) effect, other than pressure (Fig.1 A. below, first drawn for Farnsworth, 2020).
Figure
1. The informational building blocks of final cause. A) random forces
are B) constrained by form (in this case a crystalline lattice). C)
more information rich form, as in these bio-molecules can result in
e.g. ligand-receptor binding as the shapes and electrostatic fields
match (mutual information maximising) and a network of these may act as
the components of a detection-signalling pathway (D), which has
function in the context of e.g. homeostasis for the whole cell,
implying a final cause ((C) can be regarded as a magnified view of the
messenger molecule attaching to the ion channel's receptor site).
Constraints acting on forces reduce the range of directions in which forces can act among an assembly of particles. Forces can only be constrained by the relative position of the particles from which they emanate; indeed it is these positions that determine the directions in which forces act.
The positioning of the constituent parts of a system is embodied information which is effectively the form of object that we recognise from the overall assembly. When particles are positioned in a form that is not random (i.e. the information necessary to describe it is mathematically compressible), then the form has a coherent spatial structure: its spatial autocorrelation is non-zero and more generally the form has non-zero spatial mutual information (we call this `coherence') and it is illustrated by the crystal latice form in (Fig.1 B). This is the basis for effective information (Szostak, 2003). It is effective because it constrains forces in a way that gives them its coherence: specifically the directions of the forces are correlated by the mutual information of the form. The result is that forces, no longer random and merely producing pressure, act with coherence so that they are available to perform work and hence functions (e.g. the cylinder and piston of a steam engine is a form which constrains the kinetic force of steam molecules to act in a coherent direction producing a functional motion). This coherent action is nothing other than what Aristotle termed efficient cause: the action that brings about a transformation (or resists it).
Efficient cause can be interpreted as the constraint of physical forces by form: force acting under formative constraint gives efficient cause.
A nice illustrative example of this is the difference between incoherent cardiac muscle contractions (fribulation) and their coherent action that is informed by the heart's electrical impulses (proper heart contractions).
Traditional material cause, deriving from the composition of substances either acting or being acted upon by efficient cause can be seen in modern terms as a `micro-formal' cause, since it is formal cause at the atomic scale. An important example of the basic unit of efficient cause at the molecular level of material cause is the physical configuration of atoms in biologically relevant molecules that, as form, constrains intermolecular forces to act in coherent ways (coherent because there is non-zero mutual information) with effects such as molecular recognition and binding and its consequences such as conformational changes (Fig.1 C). The ability of tRNA to 'read' mRNA is a fine example: codon and anti-codon are each a form - a configuration of atoms - which correlate in space so that the electrical forces around those atoms combine to make a soft non-covalent bond (base pairing). That is quite an exotic example in the grand scheme of the universe: most material cause is simply a thermodynamically favoured configuration of atoms, such as the crystal latices of minerals such as salts and the repeated motifsfound in polymers such as cellulose.
Material cause is usefully thought of as efficent cause at the atomic level. At the next organisationally higher level, we encounter inter-molecular forms and the non-covalent bonding forces that connect several material forms (molecules) together in a functional configuration. In organisms, this can serve as an effective subsystem of biological metabolism, anabolism and perception and/or action, such as the ligand-gated channel system (Fig.1 D). There is no limit to the number of nested levels of material order (embodied information) that can be built up and all complex life arises from the information hierarchy that results.
With this in mind, we will say that efficient cause, as it is normally understood, is the product of material cause (micro-form) and information (I). This information must be embodied as form in a structure that is not transformed by the process it enacts, else it will cease to be formative. For that reason its embodying structure must be a catalyst.
Robert Rosen recognised this and expressed it in formal mathematical terms as a mapping, (Rosen,1989):
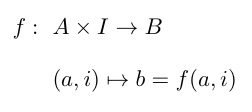
to explicitly incorporate information into efficient cause, where it plays the role of formal cause within his (M,R)-system (figure 2, taken from Cárdenas et al., (2010)). In this mapping, A mapsto B represents the set of material transformations from A to B in the (M,R)-system. The first relation is a mapping of sets, the second relation gives a general example by following the path of an individual element in the set (a in A) as it maps to b in B according to the function f which is specified by the information i as well as the source material a. Jannie Hofmayr (2007) recognised that since I is a contribution to efficient cause along with f, it should be associated with f, not A, and so rewrote this as (his Eq. 4):
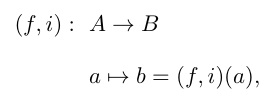
which recognises information as the formal cause that, together with efficient cause, generates the mapping (note, (f,i) is an element of {f} x I , the combination denoting i informs f, where
i and f are members of I and {f} respectively). This reformulation of mappings was developed much further by Hofmeyr (2018), where formal and efficient cause are either combined into a single entity (informed efficient cause) by a ``choice mapping'' that selects a particular f_i from a set of possible mappings, or act together as separate entities (f,i). Hofmeyr (2018) provides biochemical examples of both situations. In many biological processes, for example translation of mRNA into polypeptide, an organic code (the genetic code), instantiated as adaptors (aminoacyl-tRNAs), mediates between formal cause (mRNA) and efficient cause (ribosomes). ``Code'' is here used in the sense of Barbieri (2015): a set of arbitrary rules establishing a mapping between two independent systems, which in biological systems has the effect of "translating an organic sign into its biological meaning'' Barbieri (2015), quoted in Hofmeyr (2018). This example is illustrated on our page on Code Biology.
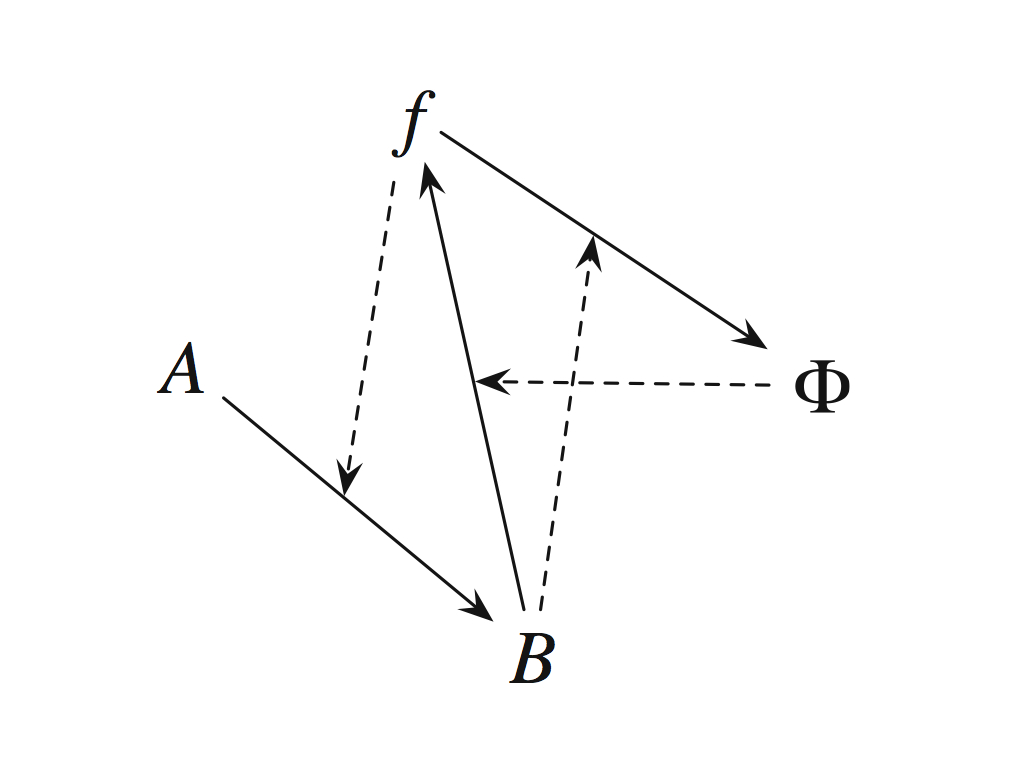
Figure 2. Rosen's (M,R)-System
redrawn to better show the reflexive nature and alternation between
material cause (solid arrows) and efficient cause (dashed arrows)
(diagram taken from Cárdenas et al., (2010). This form of the
relational diagram also helps to highlight that sources of efficient
causation act catalytically, e.g. f catalyses the material transformation from A to B.
Work and Life
Life is only possible if it can do work (in the technical sense used in physics). The textbook definition of work in this technical sense means the result of a force acting over a distance in a particular direction and you might ask what that has to do with living. The answer is that in thermodynamic equilibrium, matter consists of a lot of particles moving in random directions, hence with random momentum and this results in forces (for example gas pressure) that have no coherent pattern to them, so that on average (and we are averaging over huge numbers of particles) forces balance, so there is no net movement. This lack of net movement is a result of incoherence, of randomness and therefore of lack of pattern and therefore lack of embodied information. There is kinetic energy in all the incoherent movement, but in this random configuration, it cannot yield any work. For that we need some coherence to the momentum of the particles and this is achieved by limiting their scope, that is by constraining them (for example with a chamber and piston), which of course is equivalent to reducing the entropy of the system. Work is possible when there is a net direction to forces and this necessarily implies some organised pattern to the movements. Organised pattern, in turn, implies information. Once energy is organised into some information instantiating pattern, that pattern-information can be transferred to another component of the system. A thermodynamic work cycle (referring again to Stuart Kaufmann’s observation) is a process of extracting work from organised energy by transferring the information of organisation into another form, or part of the system. So, for example, constructing a protein molecule is an act of organising matter and to do it, life must sequester that organisation from energy. It is in this sense that catabolism (making body parts like protein molecules) uses energy. The energy is not used up (the First Law of Thermodynamics ensures that energy is never created nor destroyed); rather energy is disorganised, taking the information out of it to use in the catabolic process. The energy of photosynthetically active radiation (e.g. red and blue light) is more organised that that of heat radiation, so in transforming sunlight into warmth, plant leaves are supplying the necessary ‘organisation’ for constructing plant body parts. This ‘organisation’ is the information embodied in organic molecules: first in sugar (as a sort of currency), which is then transferred into a wide variety of molecules and structures built from them. Whenever energy is converted from a ‘high grade’ such as light to a ‘low grade’ such as heat, work can be extracted and used to construct something or perform a coherent action. Since living consists of a set of coherent and highly organised chemical reactions, many of which are also catabolic, there is plenty of need for work. Life would not exist without it.
References
Cárdenas, M., Letelier, J.C., Gutierrez, C., Cornish-Bowden, A., Soto-Andrade, J. (2010). Closure to efficient causation, computability and artificial life. J. Theor. Biol. 263, 79– 92.
Dowe, P. (2000). Physical Causation, Cambridge. Cambridge University Press, Cambridge, U.K..
Farnsworth, K.D. (2021). An organisational systems-biology view of viruses explains why they are not alive. Biosystems 200: 104324.
Hofmeyr, J.H.S., (2007). Systems biology: philosophical foundations.. Elsevier, Amster- dam.. chapter The biochemical factory that autonomously fabricates itself: a systems biological view of the living cell. Systems biology: philosophical foundations. pp. pp 217–242.
Hofmeyr, J.H.S., 2018. Causation, constructors and codes. BioSystems 164, 121–127.
Rosen, R., 1989. Newton to Aristotle. Birkh ̈auser, New York, USA.. chapter The roles of necessity in biology. pp. 11–37.
Salmon, W. C.(1984). Scientific Explanation and the Causal Structure of the World. Princeton
University Press, Princeton, USA..
Szostak, J.W., (2003). Functional information: Molecular messages. Nature 423, 689–689 doi: 10.1038/423689a.